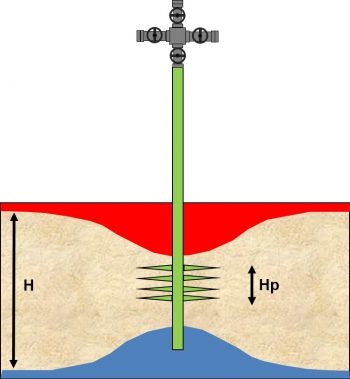
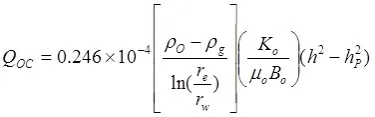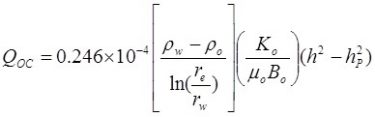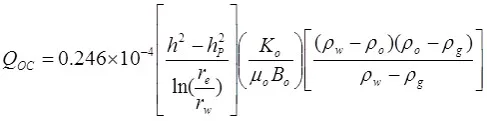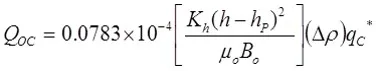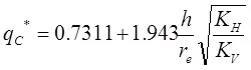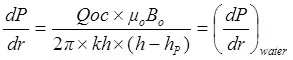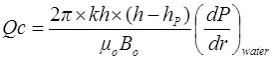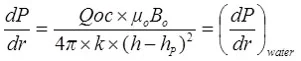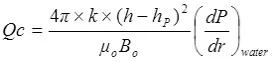Coning
Coning occurs:
-
when gas moves downwards towards the perforations of an oil well or/and
-
when water moves upwards towards the perforations.
Not only this phenomenon can impact the well performance and recovery factor, but it is also one of the “enemies” of pressure transient analysis and it should be avoided at all cost !
Viscous versus gravity forces
The problem of coning could be simplified with a problem of force balance between:
-
Viscous forces (pressure gradient that is created by the drawdown and pulls the fluids towards the perforations),
-
Gravity forces that resist coning and depend on the fluid density.
At a critical production rate, when the pressure gradient dP/dr pulling the fluid at some radius r is greater than the density gradient, coning will occur. The flowing pressure drop will become sufficient to overcome the gravity forces.
The ability and rapidity for the well to cone will depend on various factors: drawdown, vertical permeability and heterogeneity (presence of barriers to flow), distance to the fluid contact, fluid densities and viscosities, fluid saturations and relative permeability, etc…
Avoid coning at all cost !
Coning prevents the use of PTA models in a detailed analysis for well and reservoir properties. Coning will corrupt the well test data and render the PBU tests useless. As a result, it should be avoided at all cost !
-
Do not perforate close to the contact: coning could be avoided by providing a perforation standoff.
-
Slowly increase the choke by small steps and flow the well at a low drawdown, with a rate below the critical production rate. This will also help to avoid sand production and multiphase flow in the reservoir.
During well test operations, coning could be spot with an increasing BS&W or GOR during the high rate flowing periods.
Once either gas or water coning has occurred, the fluid saturation and relative permeability will increase, making coning easier to produce. It is then recommended to shut the well in and let the fluid contact re-stabilize. While this could help remove water coning, gas coning may be more difficult to eliminate, due to the increasing gas saturation.
During the design study, well testers are faced with two main problems: predict the maximum production rate and define the perforation standoff to avoid water/gas coning.
The following correlations could help.
Correlations
Several empirical correlations are available to estimate the critical production rate (max rate with no coning) and the perforation standoff.
Meyer-Gardner
Gas coning
Water coning
Gas and water coning
With
Qoc: critical production rate in stb/day
hp: perforation height in ft
h: oil column thickness in ft
μo: oil viscosity in cp
Bo: oil formation volume factor vol/vol
ρi: density of fluid i, in lb/ft3
re, rw: drainage and wellbore radius in ft
Schols
Schols developed an empirical equation based on results obtained from numerical simulator and laboratory experiments.
With
Qoc: critical production rate in stb/day
hp: perforation height in ft
h: oil column thickness in ft
μo: oil viscosity in cp
Bo: oil formation volume factor vol/vol
ρi: density of fluid i, in lb/ft3
re, rw: drainage and wellbore radius in ft
Chaperon
With
Qoc: critical production rate in stb/day
hp: perforation height in ft
h: oil column thickness in ft
μo: oil viscosity in cp
Bo: oil formation volume factor vol/vol
Δρ: difference in density in lb/ft3
Kh: horizontal permeability in mD
Kv: vertical permeability in mD
We have:
Other correlations are available in the literature.
It is worth noticing that the skin has no impact on the maximum water and gas-free production rate. Indeed, the gravity or viscous forces (pressure gradient at the fluid contact) are not affected by the skin.
Coning will be more likely the closer we are from the fluid contact with small (h-hp) values. While a perforation standoff and low drawdown will help, coning may still develop from a high permeability streak layer or as the fluid contact moves with depletion.
Example
Let’s assume an oil well with the following static and PVT data:
| Water density | ρw | 64.40 | lb/ft3 |
| Oil density | ρo | 46.80 | lb/ft3 |
| Gas density | ρg | 5.30 | lb/ft3 |
| Oil Column thickness | h | 300 | ft |
| Perforated interval | hp | 100 | ft |
| Oil viscosity | mo | 0.5 | cp |
| Oil Formation Volume Factor | Bo | 1.2 | rb/stb |
[Pressure gradient in psi/ft = (lb/ft3) / 144 ]
We want to avoid water coning and therefore “corrupting” the pressure data. Assuming a horizontal permeability of 200mD and a perforation standoff of 200ft, the maximum water-free production rate is:
| Correlation | Kv=Kh | Kv/Kh=0.1 | Kv/Kh=0.01 |
| Meyer-Gardner | 1453 stb/d | N/A | N/A |
| Schols | 2569 stb/d | N/A | N/A |
| Chaperon | 2414 stb/d | 4730 stb/d | 12054 stb/d |
Such a high perforation standoff should be considered in the well test design study as it could introduce a limited perforation effect on the derivative.
If the objective is to produce at a sustained rate of 1 mbd, how far from the contact should we perforate ?
| Correlation | Kv=Kh | Kv/Kh=0.1 | Kv/Kh=0.01 |
| Meyer-Gardner | 113 ft | N/A | N/A |
| Schols | 58 ft | N/A | N/A |
| Chaperon | 129 ft | 92 ft | 58 ft |
The correlations give different results, in part because of different assumptions.
The Meyer-Gardner and Schols should be the most conservative methods, since they assume an isotropic reservoir with Kv=Kh. A ratio Kh/Kv =1 will result in the minimum critical production rate or maximum perforation standoff. As Kv decreases, it is more difficult for the well to cone, resulting in higher critical production rate. This is well illustrated with the Charperson correlation.
Assuming a horizontal permeability K=20mD and a perforation standoff of 200ft, the results are below:
| Correlation | Kv=Kh | Kv/Kh=0.1 | Kv/Kh=0.01 |
| Meyer-Gardner | 145.3 stb/d | N/A | N/A |
| Schols | 256.9 stb/d | N/A | N/A |
| Chaperon | 241.4 stb/d | 473 stb/d | 1205.4 stb/d |
As expected, by dividing the reservoir permeability by 10, the critical production rate is divided by 10. These numbers look very conservative.
Estimating the critical production rate is a difficult problem to solve and the empirical equations may not be consistent for all the cases. For low permeability reservoirs, an approximation could be to use the Darcy law with radial or spherical coordinates to model the pressure gradients away from the perforations.
The critical production rate or the perforation standoff will then be defined when the pressure gradient equals the fluid density gradient.
Radial coordinates:
Spherical coordinates:
Download the Coning Tool !
For our VIP members, an excel file is attached to help you derive the critical production rate or perforation standoff based on the coning correlations.

only available to VIP members
Reference: “Reservoir Engineering Handbook” by Tarek Ahmed.
For more information or for a discussion, please don’t hesitate to contact us.
Avoid water or gas coning by producing the well below the critical rate and leaving a perforation standoff.
Download the Coning Tool !
This tool helps you to find the critical production rate and perforation standoff to avoid coning.